As neat lessons go, there were moments that I could see spending the time to break out play-doh to build a physical model was worth it. Students were excited to be in class (a rare treasure for Seniors about to graduate and in the midst of taking AP tests) and were able to describe what complex solid objects would look like after seeing it firsthand, instead of "imagining it" or seeing 2D computer models of 3D objects.
There were also moments that included students building snowmen or pizzas out of the building materials. But hey, did I mention how they were excited to be in class 5 weeks away from graduation? Disclaimer: I did allow 2 minutes of play time to "get playing with it out of their system" but I'm not sure that had any influence. Next time I should have a control class to test it.
This whole lesson is adapted from an activity purchased from Teachers Pay Teachers but I think next year I will adapt it to do my own thing, as well as include a student instruction sheet as the activity only had the materials. It was a great starting point to go off, however, and encourage you to go to the teacher's page to check it out. (link above) I can't link the resources here, unfortunately.
I started the lesson with a Pear Deck slide deck:
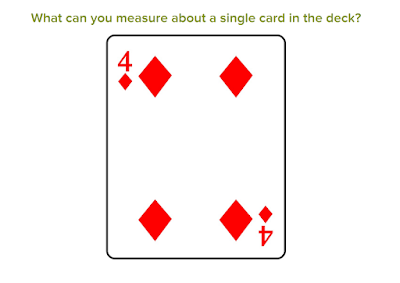
Cue: Discussion about area of a single card and off-topic but still cool discussions of flexibility, color on the electromagnetic spectrum, flammability (?!), density, malleability
Cue: Discussion about volume of the deck, and how volume of a deck is probably more relevant than volume of a single card, since cards are fairly thin
Cue: Discussion about how as the height/thickness of the card decreases, we can fit more cards into the stack. The thinner the cards, the better; we are then adding up just the area of the slices to get the volume!
At this point we stop. I don't bombard them with formulas. I had decided beforehand that my purpose of the lesson today was purely for them to gain spacial awareness of what these 3-D cross section models look like, otherwise the procedure they will eventually learn means nothing. I had thought we would have time at the end to discuss how to build a formula, but we didn't get there, so I didn't push it in an inauthentic manner just to be "able to do the homework." Next year I want to create an assignment that just has some debrief and thinking questions from the day to frontload the idea for the formula for the next.
I present them a task creating a solid using repeated shapes that we know how to take the area of, and we like the idea of adding up repeated things infinitely in Calculus. (mmm... Integrals)
They start with a set of materials:
 |
| Room layout, all materials in buckets so that I can easily grab them to prep for other class |
 |
| Play-Doh, Rulers, Scissors, Markers, Task Cards, Blank Laminated Graph Paper |
 |
| Definitely recommend Sharpies for next time, or having them draw the graph and then put it in a sheet protector |
The students work on building a solid where the base is the area under the graph of y = x^(2/3) from x = 0 to x = 8, by first graphing the function:
 |
| Featured: New scientific calculators I got from the 99 cent store (for $2) that have 2 lines of text! |
They start to add play-doh to the base, showing both the area under the graph, and allowing a surface in which to stick the cross-sections.
Note: in future years I would have them graph the function and the put a sheet protector over it for when the play-doh comes, instead of laminating them. I had them use wet-erase markers over the laminated ones and the playdoh definitely picked up some of the icky black color. Oh well! (Laminating or using a sheet protector is a must, to protect and reuse materials from class to class).
 |
| They used plastic knives to cut the play-doh to fit, and it worked really well! |
Students worked together in partners to measure the graph and construct semicircles that were the length of the function at various points on the graph. I had originally said "Make 10-15 cross sections somewhat evenly spaced out to see the 3-D shape better" thinking they could handle planning that all out. (They are 18 years old) That changed 5th period when I decided to offer a suggestion to split up making them, having one person do the whole number markings on the x-axis (1,2,3,...8) and one person to do the half-number markings (1.5, 2.5, 3.5,... 7.5) and that worked out better.
At this point, it all starts to take shape!
 |
| A mostly-finished product! |
It took much longer to finish all that then I had thought, almost 50 minutes just to set up the graph and make 1 solid with semi-circle cross sections. (I think AP students would be faster at constructing the shapes) I would set timers for each type of cross section that you do. BUT I do believe it was worth the time for them to be able to see and visualize what these models would look like, and to see the repeated shapes within them.
Ideally I wanted them to be able to "cover" their models when they were done, so they could see the smooth 3D object, but I couldn't figure out a way to do that. Another teacher had suggested colored cling wrap (which I know they sell red and green colors around the holidays) but I couldn't find any and other alternatives seemed messy and difficult.
This will now be a 2-day lesson, where Day 2 will have them continue constructing using the same base region, but different cross sections instead. (Equilateral triangles, squares, etc.) At the end of tomorrow we will develop the procedure for how to calculate the volume using Integration.
Stay tuned for Part II!





I'm totally digging this, Sarah!
ReplyDeleteIf I only had this activity when learning integrals, I actually would have remembered it (and understood) it better. Keep up the great work!